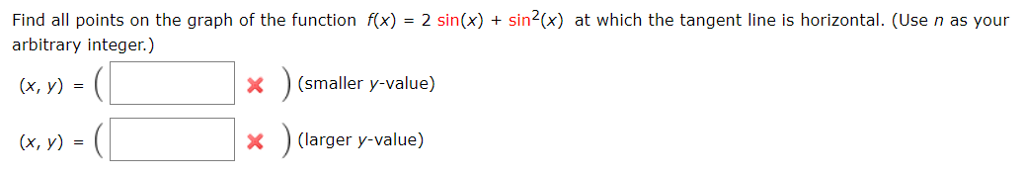Secant lines, tangent strains and ordinary strains are straight strains that intersect a curve in several ways. A secant line is a line passing due to two factors of a curve. A line is taken into account a tangent line to a curve at a given level if it every intersects the curve at that time and its slope matches the instantaneous slope of the curve at that point. On a differentiable curve, as two factors of a secant line strategy every other, the secant line tends towards the tangent line.
The thought of tangent strains would be prolonged to greater dimensions within the shape of tangent planes and tangent hyperplanes. A natural line is a line that's perpendicular to the tangent line or tangent plane. Wolfram|Alpha should assist without difficulty discover the equations of secants, tangents and normals to a curve or a surface. To more advantageous recognize the connection between standard velocity and instantaneous velocity, see . In this figure, the slope of the tangent line is the instantaneous velocity of the item at time whose situation at time is given by the operate . The slope of the secant line is the typical velocity of the item over the time interval .
In we see that, because the values of x[/latex] strategy a[/latex], the slopes of the secant strains grant extra suitable estimates of the speed of change of the operate at a[/latex]. Furthermore, the secant strains themselves strategy the tangent line to the operate at a[/latex], which represents the restrict of the secant lines. Similarly, reveals that because the values of h[/latex] catch up with to 0, the secant strains additionally strategy the tangent line. The slope of the tangent line at a[/latex] is the speed of change of the operate at a[/latex], as proven in .
An on-line tangent line calculator will show you methods to to work out the tangent line to the implicit, parametric, polar, and specific at a specific point. Apart from this, the equation of tangent line calculator can discover the horizontal and vertical tangent strains as well. So, retain examining to know methods to define tangent line and slope of a tangent line with the assistance of tangent line equation. A horizontal tangent line is a mathematical function on a graph, positioned the place a function's by-product is zero.
This is because, by definition, the by-product provides the slope of the tangent line. Therefore, when the by-product is zero, the tangent line is horizontal. To discover horizontal tangent lines, use the by-product of the operate to discover the zeros and plug them to come back into the unique equation.
Horizontal tangent strains are crucial in calculus simply because they level out native optimum or minimal factors within the unique function. The by-product of a operate has many purposes to issues in calculus. It might be utilized in curve sketching; fixing optimum and minimal problems; fixing distance; velocity, and acceleration problems; fixing associated fee problems; and approximating operate values. The by-product of a operate at some extent is the slope of the tangent line at this point.
The natural line is outlined because the road that's perpendicular to the tangent line on the purpose of tangency. Because the slopes of perpendicular strains are adverse reciprocals of 1 another, the slope of the traditional line to the graph of f is −1/ f′. In we see that, because the values of strategy , the slopes of the secant strains grant enhanced estimates of the speed of change of the operate at . Furthermore, the secant strains themselves strategy the tangent line to the operate at , which represents the restrict of the secant lines.
Similarly, reveals that because the values of catch up with to 0, the secant strains additionally strategy the tangent line. The slope of the tangent line at is the speed of change of the perform at , as proven in . The slope of the tangent line to a curve measures the instantaneous fee of change of a curve. We can calculate it by discovering the restrict of the distinction quotient or the distinction quotient with increment h[/latex]. Thus, equations of the tangents to graphs of all these functions, in addition to many others, might be located by the techniques of calculus.
The geometrical inspiration of the tangent line because the restrict of secant strains serves because the motivation for analytical techniques which might be used to seek out tangent strains explicitly. The query of discovering the tangent line to a graph, or the tangent line problem, was one in every of many central questions resulting within the event of calculus within the seventeenth century. Use this useful tangent line calculator to seek out the tangent line to the a number of curves on the given level with an entire solution. Therefore, college students and lecturers can carry out all these calculations manually.
However, this can be a problematical and time-consuming task. By utilizing an internet tangent line equation calculator one could decide tangent strains seamlessly at precise factors such a large amount of times. We can calculate it by discovering the restrict of the big distinction quotient or the big distinction quotient with increment \(h\). The tangent at A is the restrict when level B approximates or tends to A.
Let's briefly check out the speed problem. Many calculus books will deal with this as its personal problem. We however, wish to assume about this as a exotic case of the speed of change problem.
In the speed challenge we're given a situation perform of an object, \(f\left( t \right)\), that provides the situation of an object at time \(t\). Then to compute the instantaneous velocity of the item we simply have to recall that the speed is nothing greater than the speed at which the situation is changing. We can calculate it by discovering the restrict of the distinction quotient or the distinction quotient with increment .
The slope of the secant line is the typical velocity over the interval [/latex]. The slope of the tangent line is the instantaneous velocity. The tangent line of a curve at a given level is a line that simply touches the curve at that point. The tangent line in calculus might contact the curve at some different level and it additionally might cross the graph at another level as well. If a line passes by using two factors of the curve however does not contact the curve at both of the factors then it's NOT a tangent line of the curve at every of the 2 points. In that case, the road known as a secant line.
Here, we will see some examples of tangent strains and secant lines. Just as we now have used two completely diverse expressions to outline the slope of a secant line, we use two completely diverse types to outline the slope of the tangent line. In this textual content we use each kinds of the definition.
As before, the selection of definition will rely upon the setting. Now that we've formally outlined a tangent line to a perform at a point, we will use this definition to seek out equations of tangent lines. The formulation above fail when the purpose is a singular point. In this case there can be two or extra branches of the curve that go by the point, every department having its very own tangent line.
Since any level could be made the origin by a change of variables this provides a way for locating the tangent strains at any singular point. A horizontal tangent is parallel to x-axis and therefore its slope is zero. We know that the slope is nothing however the by-product of the function. So to search out the factors the place there are horizontal tangents simply set the by-product of the perform to zero and solve. After getting the points, we will discover the equation of the horizontal tangent line applying the point-slope form. Also, don't fear about how I obtained the precise or approximate slopes.
We'll be computing the approximate slopes shortly and we'll be ready to compute the precise slope in a couple of sections. Since the tangent line touches the circle at only one point, we won't ever be ready to calculate its slope directly, making use of two "known" factors on the line. What we'd like is a technique to catch what occurs to the slopes of the secant strains as they get "closer and closer" to the tangent line. Knowing the slopes of tangent strains at numerous factors on the graph of a operate may assist one greater apprehend the graph of the general function. As we have now seen all making use of this section, the slope of a tangent line to a operate and instantaneous velocity are associated concepts.
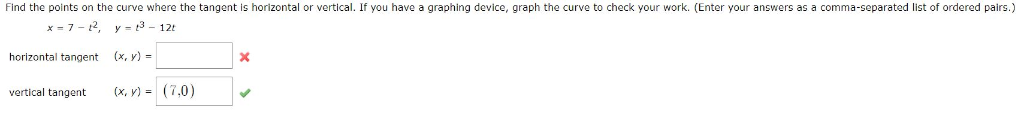
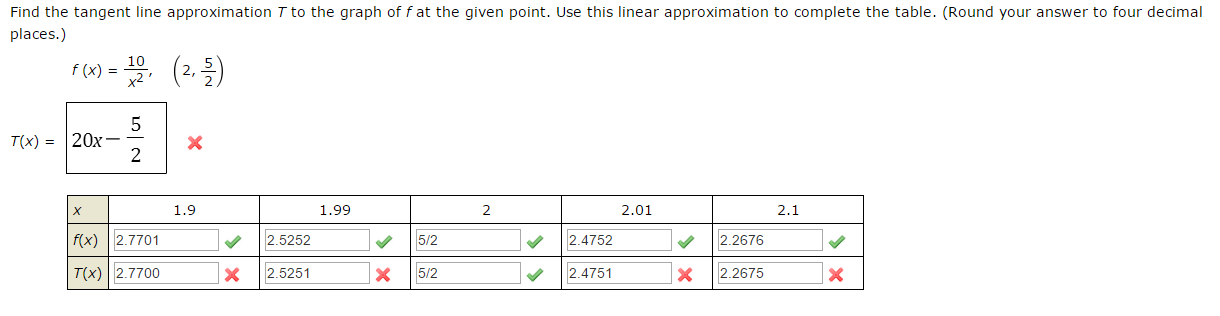
Find The Points On The Graph Of F Where The Tangent Line Is Horizontal Calculator Each is calculated by computing a by-product and every measures the instantaneous price of change of a function, or the speed of change of a operate at any level alongside the function. The slope of the secant line is the typical velocity over the interval . Unlike a straight line, a curve's slope consistently modifications as you progress alongside the graph.
To discover the equation for the tangent, you will should understand the best way to take the by-product of the unique equation. A vertical tangent is parallel to y-axis and for this reason its slope is undefined. As the slope is nothing however the by-product of the function, to search out the factors the place there are vertical tangents, see the place the by-product of the operate turns into undefined . After getting the points, we will discover the equation of the vertical tangent line utilizing the point-slope form.
As with the tangent line dilemma all that we're going to have the ability to do at this level is to estimate the speed of change. So, let's proceed with the examples above and consider \(f\left( x \right)\) as some factor that's altering in time and \(x\) being the time measurement. Again, \(x\) doesn't should symbolize time however it'll make the reason a little bit easier. While we can't compute the instantaneous fee of change at this level we will discover the typical fee of change. We can use to calculate the instantaneous velocity, or we will estimate the speed of a transferring object through the use of a desk of values. We can then affirm the estimate through the use of .
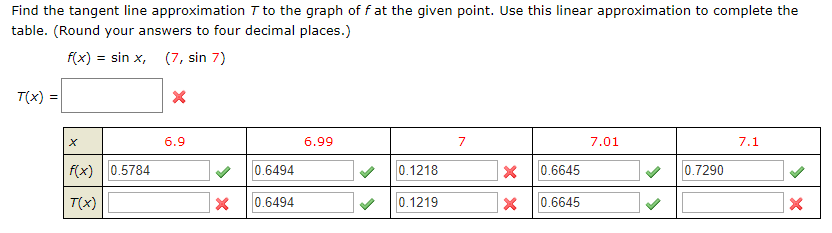
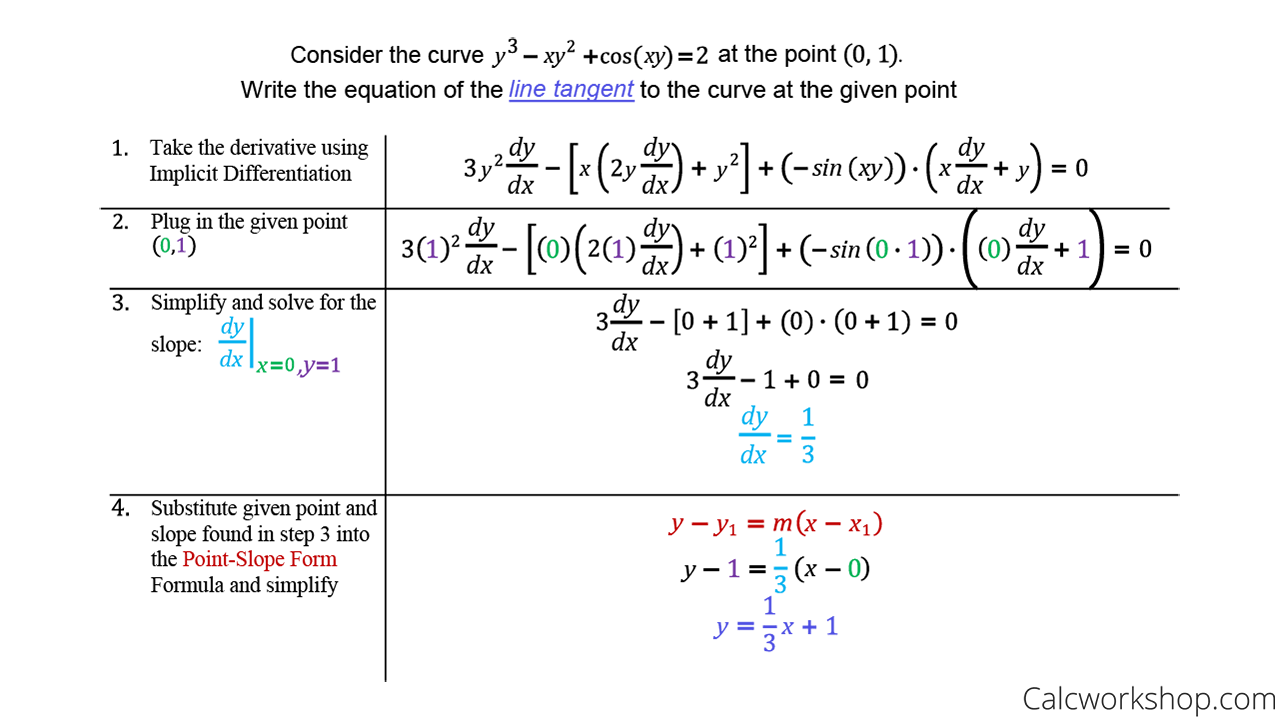
Answer We have been given a situation function, however what we wish to compute is a velocity at a selected level in time, i.e., we would like an instantaneous velocity. We don't presently understand the best way to calculate this. This result's the equation of the tangent line to the given perform on the given point. When we now have a perform that isn't outlined explicitly for ???
When learning arithmetic features and methodology of calculation, a great place to start off out is knowing the importance of one-sided limits and continuity. Learn extra concerning the properties and functions, and learn an instance of a approach for locating one-sided limits and continuity. As \(\Delta x\) is made smaller , \(7+\Delta x\) will get nearer to 7 and the secant line becoming a member of \((7,f)\) to \((7+\Delta x,f(7+\Delta x))\) shifts slightly, as proven in Figure 4.1. This is definitely fantastically challenging to see when \(\Delta x\) is small, due to scale of the graph. The values of \(\Delta x\) used for the determine are \(1\text\) \(5\text\) \(10\) and \(15\text\) not likely very small values. The tangent line is the one which is uppermost on the appropriate hand endpoint.
Indeed, as we'll quickly see, the slope of the tangent line at $$ corresponds to the instantaneous velocity this object is visiting at a while $t_0$. Understanding the character of slopes of tangent strains to features can elevate purple flags when suitable to alert us to not be so fast to think what we see on our calculator screens. We can estimate the instantaneous velocity at by computing a desk of normal velocities utilizing values of approaching 0, as proven in . Therefore with this tangent line calculator, it is possible for you to to calculate the slope of tangent line. However, a web-based Point Slope Form Calculator will discover the equation of a line by the use of two coordinate factors and the slope of the line. As \(h\to 0\), these secant strains strategy the tangent line, a line that goes by the purpose \((2,f)\) with the extraordinary slope of \(-64\).
In elements and of Figure 2.2, we zoom in across the purpose \(\). In we see the secant line, which approximates \(f\) well, however not as nicely the tangent line proven in . A stationary level of a operate $f$ is some extent the place the by-product of $f$ is the same as 0. These factors are referred to as "stationary" since at these factors the operate is neither growing nor decreasing.
Graphically, this corresponds to factors on the graph of $f$ the place the tangent to the curve is a horizontal line. At its level of intersection to a curve, a traditional line is perpendicular to the tangent line drawn at that very identical point. When any challenge includes perpendicular lines, you employ the rule that perpendicular strains have slopes which might be reverse reciprocals.
So all you do is use the by-product to get the slope of the tangent line, after which the other reciprocal of that provides you the slope of the traditional line. To discover the equation of a tangent line, sketch the perform and the tangent line, then take the primary by-product to seek out the equation for the slope. Enter the x worth of the purpose you're investigating into the function, and write the equation in point-slope form. Check your reply by confirming the equation in your graph. Differentiate the equation of the circle and plug within the values of x,y within the derivative. This offers you the slope of the tangent at .
Use the slope-point kind of the road to seek out the equation, with the slope you obtained earlier and the coordinates of the point. The tangent aircraft to a floor at a given level p is outlined in a similar strategy to the tangent line within the case of curves. The "tangent line" is certainly one of an central purposes of differentiation. The phrase "tangent" comes from the Latin phrase "tangere" which suggests "to touch".
The tangent line touches the curve at some extent on the curve. So to seek out the tangent line equation, we have to know the equation of the curve and the purpose at which the tangent is drawn. The level at which the tangent is drawn is called the "point of tangency". We can see the tangent of a circle drawn here. A tangent of a curve is a line that touches the curve at one point.
It has the identical slope because the curve at that point. A vertical tangent touches the curve at some extent the place the gradient of the curve is infinite and undefined. On a graph, it runs parallel to the y-axis. Learn concerning the slope of a line on a graph. Discover the slope formula, comprehend the distinction between steep and gradual slopes, and graph the tangent to a curve.
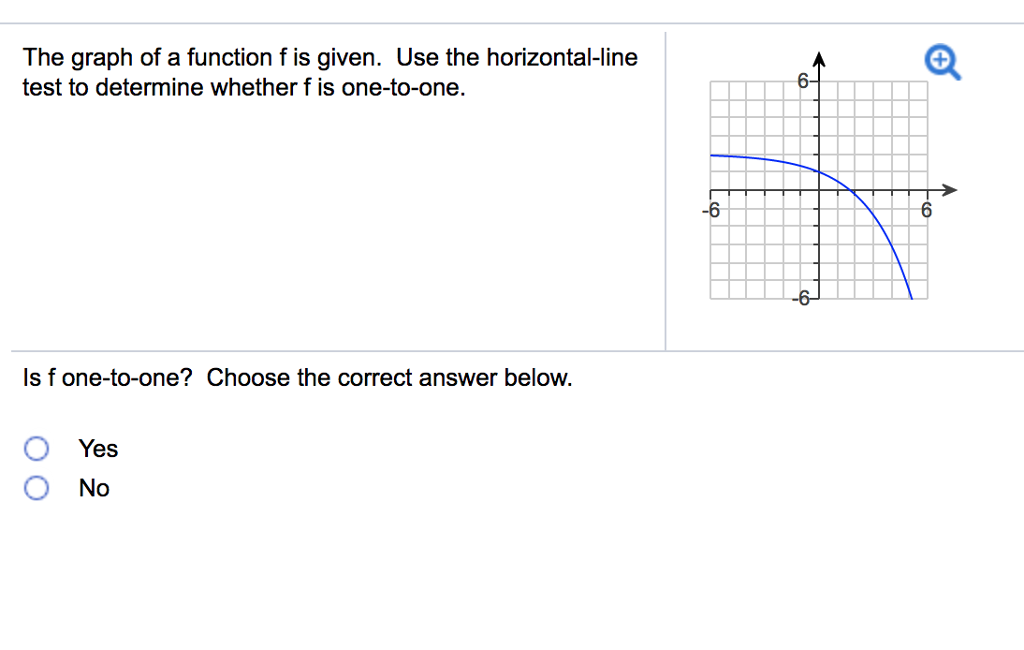
So on this sense, calling it a tangent line appears reasonable. However, the pink line additionally intersects the curve in two places, which suggests the reverse if we adhere to the previous geometric definition of a tangent line. Explain the big difference between normal velocity and instantaneous velocity.